Where does the exp come from?

It is something of a mystery how the formula for compound interest changes its form so completely.

Unfortunately the reason depends on some fairly technical looking mathematics. You don’t have to understand the contents of this section to make use of the exponential functions but it is interesting.

The Future Value of an investment earning% per annum compounded n times per year is:

(1+I/n)^(t*n)

where t is the fraction of a year that has passed.

For example, if n is 365, i.e. daily compounding then the future value after half a year is:

(1+I/365)^(0.5*n)

If n is increased the number of compounding periods increase and the larger n becomes the closer we approach continuous compounding. In mathematical terms we need to investigate the form of the equation as n, the number of compounding periods per year, tends to infinity.

It turns out to be easier find the limit in terms of the new quantity n’ which is the number of compounding periods divided by the interest rate that is

n’=n/I

and so

n=n’*I

Rewriting the formula in terms of n’ gives:

(1+1/n’)^(n’*I*t)

Now you can see the advantage of the new quantity n’ because we can reduce the problem to finding the limit of:

(1+1/n’)^n’

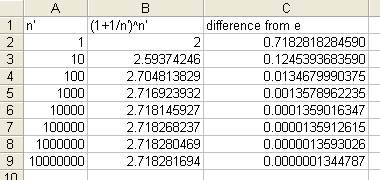
as n’ goes to infinity independently of the interest rate and the time interval.

You can investigate this quantity as n’ gets bigger using a spreadsheet and you will find that it tends towards a value of 2.7182818285 which is known as the exponential number.

For example, in the spreadsheet below you can see a table of values of (1+1/n’)^n’ for large, but certainly not infinite, values of n’. Notice how the value gets ever closer to the constant e. How far you can continue this process depends on the largest number that the spreadsheet you are using can cope with and on the accuracy of the calculation.

Given that the limit of (1+1/n’)^n’ is e, the formula for the future value at any time t is simply,

FV=PV*e^(t*I)

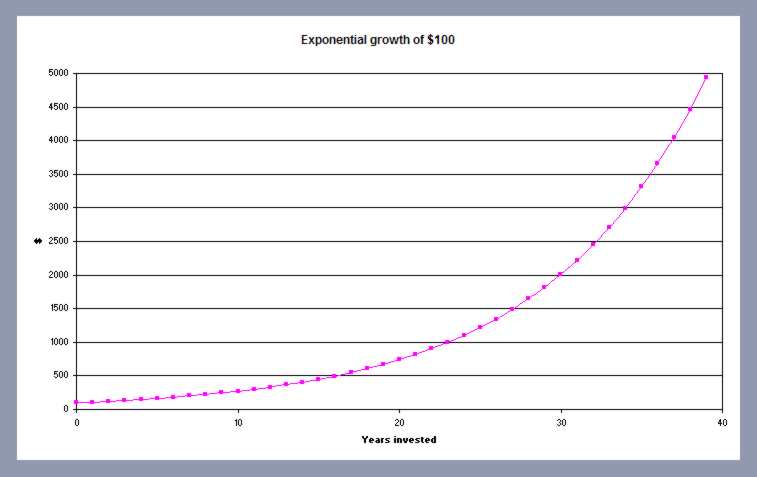
You can see the effect of continuous compounding on $100 at 10% per annum in the chart below:

To create this chart with $100 as the present value and 10% as the interest rate over a period of 40 years simply enter a series in column A from 0 to 40 years starting in A2, enter the formula:

=100*EXP(A2*0.1)

in B2 and copy it down the column to B42 and then create an XY chart of the area A2:B42.

Notice the characteristic way that the rate of increase is itself increasing.

Once you have the formula for the Future Value working out the effective annual rate under continuous compounding is simple and gives:

effective rate = EXP(I)-1

Net and gross - tax allowances

If interest is paid on a deposit then tax is usually payable on the income. Normally interest rates are quoted gross, i.e. without taking tax into account.

For example, if the deposit of $100 earns 10% effective per annum then $10 is earned in the first year, but T% of this has to be paid in tax, i.e. reducing it by T%.

Thus the interest actually received at the end of one year is:

tax paid interest=PV*I*(1-T)

You should be able to see that the effect of having to pay tax reduces the effective rate from I% to I*(1-T)%.

An interest rate that doesn’t take tax into account is called a gross rate and one that does is called a net rate.

To convert from gross to net use the formula:

net = gross*(1-T)

to convert from net to gross use:

gross=net/(1-T)

For example, if a bank offers a gross rate of interest of 4% and tax is paid at 20%, then this is equivalent to receiving 0.04*(1-0.20) or 3.2% gross.

There is always the question of which rate should be quoted in connection with an interest bearing investment - gross nominal, net nominal, gross effective or net effective.

The financial institution always prefers to quote the highest possible rate and this is, of course, the effective gross rate, also called the gross CAR (Compound Annual Rate).

In all situations the net nominal rate should be converted to the net effective rate before being converted to the gross effective rate or gross CAR.

Of course what matters to the investor is the net effective rate because this reflects what is actually received on the investment. However, in comparing interest rates all that really matters is that the same type of rates are compared.

Calculating and compounding

There is one last complication to be considered.

When a balance on deposit can vary from day-to-day calculating the interest to be added for a longer compounding period presents something of a problem.

For example, suppose a bank current account offers 1% per month with interest added monthly - what balance should be used to calculate the monthly interest?

As the balance can change each day it seems more reasonable to calculate the interest daily using a rate of I%/365 but only add the interest once a month.

This is how nearly all bank accounts work, including overdrafts, with interest calculated on the balance daily but only being added monthly or at a lesser frequency. (Of course the interest rate for an overdrawn account will be different from the one applied to one in credit.)

This doesn’t alter any of the calculations described earlier and the effective rate is still given according to the compounding period and not according to period used to calculate the interest.

It is only when the interest is added to the account that matters. If the balance in the account is constant then the daily calculation of interest produces the same result as calculating and adding the interest at the end of each compounding period.

In the case of banks, the daily balance may not be what appears on the statement. The reason is that a cheque is credited to your account when it is paid in but only cleared for interest calculations three or so working days after that.

Trying to keep an exact check on a daily bank balance can be very tricky as only the bank will actually know when a cheque has cleared for interest.

This use of daily interest calculation also raises the question of how many days there are between any two given dates. Dates and date arithmetic is the subject of a later chapter.

<ASIN:/0230348114>

<ASIN:1118510100>

<ASIN:0735672431>

<ASIN:0789748576>