| Simple and Compound Interest - Time Is Money |
Page 1 of 5
Chapter 2We explore the idea of borrowing money for a specified rate of interest or earning interest on an investment.
Financial Functions
Buy from AmazonSpreadsheets take the hard work out of calculations, but you still need to know how to do them. Financial Functions with a spreadsheet is all about understanding and reasoning, using a spreadsheet to do the actual calculation.
<ASIN:1871962013> <ASIN:B07S79ZVMQ> The idea of borrowing money for a specified rate of interest or earning interest on an investment.is something that we are all familiar with. Interest is a percentage, but one that has a time-based component. Interest is calculated and paid at a regular intervals and this makes its behaviour rather more varied than a simple static percentage. Interest - a percentage rateMany financial arrangements are specified in terms of interest which is a percentage of the total per time period. Interest is a percentage rate - so many percent per month, so many percent per year and so on. It is a rate in the sense of something that involves the passage of time - miles per hour, kilometres per second and 10% per month are all rates. In the days before legislation tightened up on how interest rates were quoted, it wasn’t uncommon to find quotes of 10% interest, but without any mention of the time period involved - and 10% per day is a very different amount of money from 10% per annum. Thus there are two important components of any interest specification:
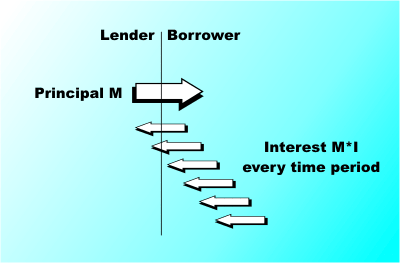
This view of percentage as a rate makes clear some of the difficulties in store for us. For example, if you can make a return of 1% per month, 3% every quarter or 11% per annum, which is the better investment? A small bank loan is offered at 20% per annum, but a credit card load costs only 2% per month which is better? Clearly converting between interest quoted for different time periods is something that we are going to have to examine. But first we need to look at the way that interest is calculated. Lenders and borrowersInterest is paid on deposits and charged on loans. These two situations are in fact identical from the point of view of calculating interest. In each case there is an investor/lender who provides the lump sum - the principal - and a borrower who pays interest on the loan/investment. It doesn’t really matter if the borrower is in fact called a bank, an investment trust or John Smith, the cashflows are the same.
If the principal is $M and the interest rate is I% the interest due each payment period is simply:
Notice that we are not considering the repayment of the loan nor the accumulation of interest. If the principal is a loan then it is assumed that the whole principal will be paid back as a lump sum sometime in the future - i.e. it is an interest only loan. If the principal is an investment then the interest is paid out to the investor and not reinvested. The key factor is that the interest is paid in such a way that the value of the principal, i.e. $M, remains constant over time. In this case the amount of interest paid in each time period is also constant and this results in a very easy to manage situation - simple interest. Present and Future ValueIt is common practice to use the terminology Present Value, or PV, for the sum of money involved at the start of a loan or investment and Future Value, or FV, for the final balance. In other words, FV is what results after interest has acted on PV. This jargon applies equally to investments or loans:
Other terms, such as principal, are used for PV but for the remainder of this book PV and FV will be used to denote the value before and after the action of interest respectively. Notice that the relationship between PV and FV depends on the type of situation we are considering. For example, in the case of simple interest of I% over n interest bearing periods the FV is given by:
or:
You should be able to recognise this as just increasing the PV by I*n%. Comparing simple interestIn the situation where interest is paid on a PV that does not change over time, it is very easy to compare different interest rates. For example, if a deposit pays 2% interest per month then over a 12-month period the total amount paid in interest is simply:
or:
This implies that to receive 2% per month is equivalent to receiving 24% per annum. This same reasoning applies to any interest rate over any time period.
For example, 10% paid every six months, i.e. two interest bearing periods per annum, is equivalent to a rate of 0.10*2, i.e. 20% per annum. In other words, for simple interest rates converting between different periods really is just a matter of multiplying by the ratio of the periods. For example:
Notice that for all of these examples to be correct the situation must correspond to simple interest, i.e. the interest calculated is not added to the PV. <ASIN:/0230348114> <ASIN:1118510100> <ASIN:0735672431> <ASIN:0789748576> <ASIN:0470242051> |